Barbara Thompson's 1995 AGU Spring Meeting Poster
Alfven Wave Interactions
Basic Introduction - What is an Alfven wave?
An Alfven wave is like a wave travelling along a stretched
string. The magnetic field line tension is analogous to
string tension, and when the magnetic field is "plucked"
by a perturbation, the disturbance propagates along the
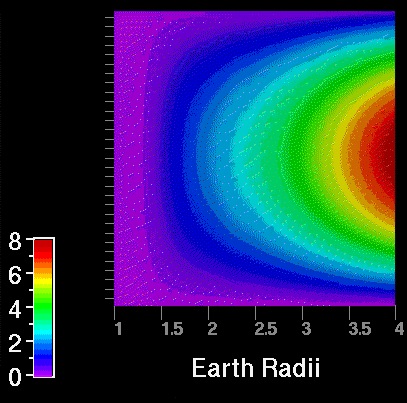
field line. At auroral altitudes, an Alfven wave typically
has frequencies near a few Hertz. This corresponds to wavelengths
reaching an Earth radius - this long-scale coherence, coupled
with the notion that the wave is carried by ions and is capable
of transporting significant energy in the form of
Poynting flux towards the earth, indicates that Alfven waves
may play a significant role in magnetosphere-ionosphere coupling.
Derivation of Wave Equations (sort of)
In addition to a standard Alfven wave, the effect of electron
inertia is included (see equation on Electron Inertia page in
poster). For conditions in auroral regions, the inertial term
reduces to a simple expression for the parallel electric field.
The current is related to the vector potential through the
magnetic field, resulting in an expression for the parallel electric
field in terms of the time derivative of the vector potential.
The overall electric field is related to the vector and scalar
potentials by Faraday's law (the gradient of the scalar
potential plus
the time derivative of the vector potential).
The Alfven wave is expressed in terms of its vector and scalar
potentials. This is accomplished by treating it as a wave in a
dielectric medium, with the
dielectric constant and the magnetic permeability expressed in
terms of the plasma density and the background magnetic field.
Since the parallel electric field due to the
electron inertial effect becomes another term depending on the
vector potential, the equations continue to depend on the
vector and scalar potentials, and the two functions are integrated
together.
Dielectric treatment of Alfven waves
The dielectric constant of an Alfven wave is e = 1 + c^2/VA^2,
where
VA is the Alfven speed and c is the speed of light. This relates
the electric field and displacement field, E and D. The
magnetic field and magnetic inductance have a similar relationship.
After fourier transforming in the direction perpendicular to the
geomagnetic field (assuming that most of the variation is along
the field lines) and examining the equations, it becomes apparent
that a factor u = 1 + Kperp^2lambda^2 exists where the magnetic
permeability would be in Maxwell's equations (Kperp is the
perpendicular wave number and lambda is the electron inertial
length, c/w, where w is the electron plasma frequency.)
Since u = 1 + x, where x is the magnetic
susceptibility, we conclude that x = Kperp^2lambda^2
is the effective magnetic susceptibility of an inertial Alfven
wave. The
factor u = 1 + Kperp^2lambda^2, like the dielectric constant, is
also a function of the background geomagnetic field and plasma
density. As e = 1 + c^2/VA^2 relates the fields E and D,
u = 1 + Kperp^2lambda^2 relates B and H.
Because we have expressions for both the magnetic permeability
and the dielectric constant, we can solve for the index of
refraction: n = sqrt(ue). This is equal to the speed of
light divided by the phase
speed of an electromagnetic wave in the medium. Solving for the
phase speed, we see that electron inertia slows an Alfven wave
down by a factor of 1/sqrt(u). Further derivations reveal
that u reduced field line tension, and affects the
equations for Poynting flux and diffusion.
Return to
Summary Page
Other Sections
System properties and physical behavior
Wave integration - fluid motion
Electron integration - particle motion
Electron effects and commonly observed
distributions
Conserving energy -
numerical scheme
Chaotic behavior of system
References - please see poster