MAGNETIC MOMENTS, Number 8
ANTENNA NOISE
(originally printed in Speleonics 11, Nov 1988, p6)
By Ian Drummond
The last "Magnetic Moments" had a discussion of the natural sources of noise in the atmosphere, and showed how the noise field strength in micro-amps/metre could be estimated for a given place and time on the Earth's surface.
A second source of noise which obscures the desired signal is the receiving antenna itself. Any physically real antenna has electrical resistance and there is thermal noise associated with that resistance.
A.D. Watt deals with this problem in "VLF Radio engineering", Pergamon Press, 1970. and derives the following relationship for air-cored loops, transformer coupled to the receiver.
| H(noise) = | -27.9 -10log f -15log A +10log K -10log Q +10log B + Nt + Fv |
| (dB rel. 1 microamp/m) | |
|
where: f = |
frequency (Hz) |
| A = | area of loop (m2) |
| K = | induction factor (fig. 1) |
| Q = | antenna Q factor |
| B = | receiver bandwidth (Hz) |
| Nt = | transformer degredation factor (dB) |
| Fv = | Receiver noise factor (dB) |
The noise field of the antenna can be compared to the atmospheric noise field to determine which is predominant. For small loops sometimes antenna noise can in fact exceed the atmospheric noise. Thus a larger (and thermally quieter) antenna could improve system performance. Once the optimum size is reached, however, no increase in the receiver system performance will result simply from a larger antenna; that is, the signal-to-noise ratio at the receiver input will not be increased by a larger antenna.
For example the noise field of the small ASS cave radio antenna can be compared to the atmospheric noise found in Alberta.
| Constant = | -27.9 | ||
| f = | 115400 Hz | -10log f = | -50.6 |
| A = | 0.5 m2 | -15log A = | + 4.5 |
| K = | 2.8 | +10log K = | + 4.5 |
| Q = | 80 | -10log Q = | -19.0 |
| B = | 1500 Hz | +10log B = | +31.8 |
| Nt = | 4 dB | (assumption) | + 4.0 |
| Fv = | 3 dB | (assumption) | + 3.0 |
|
| |||
|
-49.7 dB (rel 1 microamp/metre) |
In Magnetic Moments #7 the noise field strength at 115.4 kHz in a bandwidth of 1500 Hz, on a summer afternoon in Alberta, was derived as -39 dB(1microA/m). Thus under these conditions the antenna noise field is 10 dB below the atmospheric noise and (quite by accident) the receiving antenna would seem to be about the optimum size! In fact Alberta can be significantly quieter at other times and a larger receiving antenna can be beneficial in extending range under these circumstances of lower atmospheric noise.
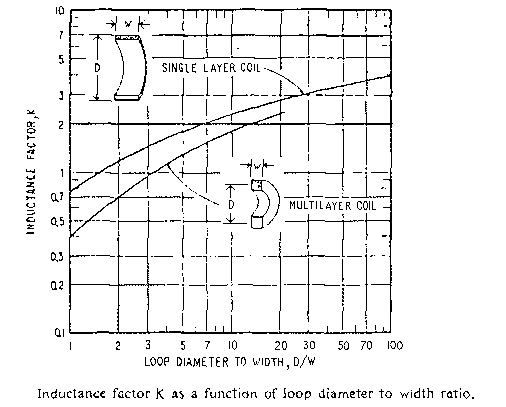
Figure 1. Inductance of coil = K.A0.5.N2 microHenries
Return to the Top of the Page
Copyright © 2000 Communications & Electronics Section of the NSS, Inc. - All Rights Reserved.