Modern Amplifier Terms Defined
1 dB compression point defines the output level at which the amplifier's gain is 1 dB less than the small signal gain, or is compressed by 1 dB.
Conditionally stable amplifier refers to an amplifier which will oscillate under particular load or source impedance (VSWR) conditions, an undesirable situation.
Cp (Process capability) Process capability
broadly defined as the specification width (S) divided by the
Process width (P) and is an indication of the spread of the
process. Specification width "S" is the difference
between the upper specification limit (USL) and the lower
specification limit (LSL). Assuming the process to be Gaussian,
its standard deviation can be denoted by sigma(
). Process
width is defined as 6 times sigma (3 sigma on each side of mean).
For example, if the USL and LSL of noise figure of an amplifier
are 6.9 and 6.0 dB, then S is 0.9 dB. If the standard deviation () is 0.1
dB, then P is 0.6 dB. Cp, the process capability, is 0.9/0.6 and
is 1.5.
When Cp is 1, then 99.73% of the units pass specs and the
process produces 0.27%. rejects. When the value of Cp increases,
the number of rejects reduce dramatically. Percent defects are no
longer used at higher values of Cp, instead parts per million
(ppm) is used to describe the number of rejects. For example,
when Cp is 1.5, rejects are 5 ppm and when Cp is 2 the rejects
are 0.002 ppm (In this case process width is ± 6 and the
process is called a 6process). All the above numbers are based
on the assumption that the center of the spec limits and the
center of the process are the same. When this not true, Cp does
not provide complete information.
Cpk (Process capability of a non-centered process)
Cp does not take into account non centering of the process and
hence is of minimal value in practice. In this case another term,
called Cpk, is used and takes into account any non-centering of
the process and is defined as
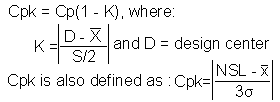
where NSL is the nearest spec limit, 
is the mean of the
process, and the vertical lines indicate that Cpk is always a
positive number. Cp and Cpk are equal for a centered process. Cpk
is also useful for defining processes with single-sided specs.
For example, noise figure of an amplifier has only an upper spec
limit and active directivity a lower spec limit. In deriving Cpk,
one should make sure that has a meaningful value, such as between
spec limits when both spec limits are present. For single-sided
specs,
should be below the upper spec limit or above the lower spec
limit. The graph below shows the number of rejects for various
values of Cpk.
Directivity (active) is defined as the difference between isolation and forward gain in dB. It is an indication of the isolation of the source from the load, or how much the load impedance affects the input impedance and the source impedance affects the output impedance. The higher the active directivity (in dB), the better the isolation.
Dynamic range is the power range over which an amplifier provides useful linear operation, with the lower limit dependent on the noise figure and the upper level a function of the 1 dB compression point.
Gain flatness indicates the variation of an amplifier's gain characteristic over the full frequency response range at a given temperature expressed in dB.
Gain (forward gain, G) for RF amplifiers is
the ratio of output power to input power, specified in the small
signal, linear gain region, with a signal applied at the input.
Gain in dB is defined as, GdB = 10 log10 G.
Harmonic distortion is produced by nonlinear amplifier operation and appears in the form of output signal frequencies at integral multiples of the input signal frequency. Since harmonic distortion is influenced by input power level it is generally specified in terms of the relative level for the harmonics to the fundamental signal power.
Isolation is the ratio of the power applied to the output of the amplifier to that measured at the input of the amplifier.
Linearity of an amplifier signifies its output power is a linear function at the input power. A linear amplifier produces at its output an amplified replica of the input signal with negligible or no harmonic generation.
Maximum signal lever refers to the largest CW or pulse RF signal that can be safely applied to an amplifier's input. Exceeding the specified limit can result in noise figure degradation, increased distortion, gain reduction, and/or amplifier burnout.
Noise factor is the ratio of signal-to-noise power ratio at an amplifier's input to the signal-to-noise power ratio at the output. Noise figure NF in dB is related to noise factor F by
NF = 10 log10F in dB
Return loss(RL) is the ratio of reflected
power to incident power at the RF port of an amplifier, expressed
in dB by return loss (RL = -20 log |
|, where = reflection coefficient.
Reverse gain is the ratio of power measured at the input of an amplifier to the applied power at the output of an amplifier, also known as isolation.
Sigma is the statistical term for the
Standard Deviation of a distribution. All things differ one from
another to a greater or lesser degree. The measure of how much a
distribution varies around the average or middle valueis a measure
of its standard deviation. Most distributions take a bell-shaped
curve appearance, called a normal distribution. The distance from
the center of a normal distribution curve to where the curve
changes direction, or its point of inflection, is called the
standard deviation or the Greek letter sigma; one sigma denotes
one standard deviation.
Sigma performance, SP, tells how far the nearest spec limit is from the average, compared with the sigma value. For example, if the spec limit is 6 sigma away, the process has SP = 6. Thus, 5P equals 3 times the CpK value.
"Skinny" sigma refers to a small deviation. The relationship between the process standard deviation and the width of the specification reflects the sigma. For example, in a well-controlled process the nature of deviation from one unit to the next is small and most units thus fall well within the spec limits; the narrow variation indicate a "skinny" sigma. In a process that is not tightly controlled, units will vary from one spec extreme to the other or even exceed the spec limits; here the standard deviation and sigma are wide. What causes some confusion is the fact that 6 sigma is skinny while 2 sigma is wide; 6 sigma means the spec limits are much further away from the distribution.
Stability of an amplifier is an indication of its tendency to oscillate or generate a signal at its output without an applied input.
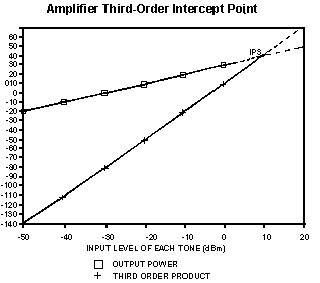
Two-Tone Third-order intercept point Two-tone third-order intercept point is a measure of third-order products generated by a second signal arriving at the input of a device such as an amplifier. If F1 and F2 are the frequencies of the two signals arriving at the input, the amplifier generates intermod products at its output due to inherent nonlinearity, in the form ± m*F1 ± n*F2 where m and n are positive integers which can assume any value from 1 to infinity. The order of the intermod is defined as m + n. Hence 2*F1 F2, 2*F2 F1, 3*F1 and 3*F2 are third-order products by definition. The first two products are called two-tone third-order products as they are generated when two tones are applied simultaneously at the input and the latter two are called single-tone third-order products.
For example, if 100 and 101 MHz are the frequencies of two applied signals, then 99 and 102 MHz are the two-tone third-order products and 300 and 303 MHz are single-tone third-order products. Two-tone third-order products are very close to the desired signals and are very difficult to filter out. Hence they are of great importance in system design.
In the linear region, third-order products decrease/increase by 3 dB for every 1 dB decrease/increase of input power, and output signal power decreases/increases by a dB for every dB of input power. When drawn on a X-Y graph, with input power on X-axis and output power on the Y-axis, third-order products fall on a straight line with a slope of 3 and signal power on a straight line with a slope 1 as shown below. By extending the linear portions the two lines, they intercept at a point. The X co-ordinate and the Y co-ordinate of this point are called the input and output intercept point, and the two differ by an amount equal to the small-signal gain of the amplifier. Output intercept point, IP3(dBm) can also be calculated using a simple formula.
IP3(dBm)out = Pout(dBm) + A/2
where Pout (dBm) is the output power of each tone in dBm and
"A" is the difference of output power and intermod
level in dB. Input intercept point is obtained by substituting
Pin(dBm) for Pout(dBm) in the above formula. Single-tone and
two-tone third-order intercept points differ by a fixed amount
but have the same slope.
Unconditionally stable refers to an amplifier
that will not oscillate regardless of load or source impedance.
VSWR (voltage standing wave ratio) is related
to return loss(RL) by the following:
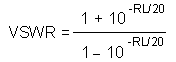
Q. What is the effect of using a 50-ohm amplifier in a 75-ohm system?
A. When a 75-ohm load is seen from an ideal 50-ohm amplifier or vice-versa, a 1.5:1 VSWR results which alters gain, output return loss, and gain flatness in real-life amplifiers. If active directivity (defined as isolation minus gain) is low, a change in load impedance will result in a change of input impedance and a change in source impedance will result in a change in output impedance. Hence, a 75-ohm load on an amplifier with low directivity will affect the input impedance of an amplifier. Maximum transfer of power may not occur.
However, in many applications, the mismatch may not be objectionable. For specific performance details, the 50-ohm amplifier should be tested under 75-ohm conditions. Contact the factory or sales rep. for computer automated performance data (CAPD) on the amplifier you are considering.
Q. What is output VSWR and what is its significance?
A. Output VSWR is a measure of how much power is reflected back from the amplifier's output port when an external signal is applied to that port. VSWR varies from a theoretical value of 1:1 for a perfect match to greater than 20:1 for total mismatch. Since loads in practical applications vary with frequency, maximum power and gain flatness also will deviate from what is specified. If the amplifier is connected to its load by a cable and all three have different impedance, then multiple reflections between the amplifier and its load can occur resulting in greater variation in frequency response. In general, the output impedance (characterized by output VSWR) is the source impedance of the following device.
Q. How is output VSWR measured?
A. A simple setup using a directional coupler is shown below.
First establish the 0 dB reference as follows. Apply the input signal to the directional coupler output port as shown. Apply a short circuit to the coupler's input port and measure the power at the coupled port. Then replace the short with an open circuit and note the reading at the coupled port. The average of the two readings is the 0 dB reference. Next substitute the open circuit with a 50 ohm load. Note the reading; this will give you the measurement range of the setup. Remove the 50 ohm load and replace it with the DUT. Measure how far the reflected signal is from the 0 dB reference; this is the output return loss (R.L.). To convert output return loss to VSWR, use the formula:
For more accurate measurements, use a scalar or vector network
analyzer.
Q. What is the relationship between reflection coefficient, VSWR, and output return loss?
A. The voltage
reflection coefficient () is the ratio of the reflected to
incident voltage in an amplifier or device. The theoretical
reflection coefficient varies from zero for a perfect match to
one for a total mismatch. Magnitude of reflection coefficient and
VSWR are related by
Return loss is related to the magnitude of reflection coefficient
by
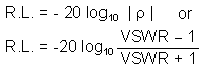
Q. To improve matching, can I
use a resistive pad between amplifier stages?
A. Of course.
But at the expense of overall gain, noise figure, and/or output
power. The higher the gain of the first stage and the lower the
value of the attenuator, the less the degradation of noise
figure. Overall noise figure is calculated as follows:
Where F1, F2 are noise factors of first, and second amplifiers,
and L is the loss of the pad.
Noise figure in dB = 10 log10 F, where F is noise
factor
Loss in dB = 10 log10L, where L is loss factor
Gain in dB = 10 log10G, where G is gain factor
Q. What is the significance of an amplifier's directivity characteristic in a system design?
A. Directivity
is the difference between isolation and gain. Directivity is an
indication of how the impedance mismatch at the amplifier's
output affects the input.
In receiver applications, a filter following a wideband amplifier
reflects undesired signals back to the amplifier and then to the
mixer stage. If the amplifier provides high directivity, the
reflected signals reaching the mixer will be much lower in
magnitude and thus have little inter-action at the mixer stage.
Another common application is two-tone, third-order IM testing, where the two-tone signals must be well isolated; amplifiers with high directivity are used between source and combiner.
For relatively high RF frequencies, isolators can be used but they are expensive; for frequencies below 1 GHz, they are difficult to find.
High-directivity amplifiers, such as Mini-Circuits' MAN-AD series, are recommended for such applications.
Q. Can I obtain higher power output by paralleling amplifiers?
A. Yes, but it's not as simple as merely connecting the two inputs and the two outputs in parallel. It involves judicious use of power hybrids with proper amplitude and phase balance and power levels, as well as amplifiers well matched for gain and phase characteristics. Quite candidly, it's generally advisable to specify an amplifier that meets the high-power requirements.
Q. I want to vary an amplifier's gain. Can I adjust the amplifier's supply voltage to achieve an AGC effect?
A. It's not recommended. An amplifier is designed to operate at a given supply voltage and its performance specifications (gain, power output, saturation, frequency response, etc.) are based on the stated supply voltage. Boost the supply voltage too much and gamble on the amplifier burning up; reduce the supply voltage too low and expect the performance specs to deviate considerably.
Variable-gain amplifiers are available, such as Mini- Circuits' ZFL-1000G and ZFL-1000GH, to accomplish your objective.
Q. I'm working with a 12-volt system and am considering your +30 dbm ZHL-series amplifiers. The specs indicate a +15 volt supply is required. How will performance be affected with 12-rather than 15-volt DC input?
A. Mini-Circuits' RF Signal-Processing Handbook, Vol. 3 on Amplifiers provides performance figures for three DC power input levels: +15, +16, and +12 volts. Need a copy? Ask your local Mini-Circuits' sales representative or contact the factory for a free copy.
Q. Is there a simple way to estimate second- and third-order intercept point for an amplifier?
A. As a general rule, the second-order point is 18 to 20 dB above the 1 dB compression point while the third- order intercept point is 10 dB above the 1 dB compression point.
Q. My application involved injecting sharp RF pules to an amplifier. How can I tell whether the amplifier's peak power limits will be exceeded?
A. Here's a conservative rule-of-thumb estimate for a 50-ohm amplifier. (1) Take the amplifier's maximum power spec. (2) Convert from dBm to W. (3) Multiply by 100. (4) Take the square root, and use this figure as the maximum peak voltage that can be applied.
Q. When an amplifier is used in a test setup, is there such a thing as a safe sequence to connect the amplifier's input, output, and supply voltage to avoid damage?
A. Yes, there is a recommended procedure. Begin by connecting the load, then the DC supply, and finally the RF input signal. When finished, first disconnect the RF input, then the DC power, and finally the load.
Q. Please sketch the test setup and describe the test procedure used to measure an amplifier's second-order intercept point.
A. Block diagram of a set up for measuring amplifier harmonics is shown below. Second harmonic of an amplifier is quantified and specified as second order intercept point (IP2). In the linear region of the amplifier, if second harmonic is A2 dB below fundamental, this IP2 is given by
IP2(dBm) = Pout (dBm) + A2
In the block diagram, a low-pass filter is provided to
attenuate second harmonics of the generator 10 to 20 dB below
that generated by the amplifier. Sufficient attenuation should be
provided at the amplifier output to prevent spectrum analyzer
from generating harmonics.
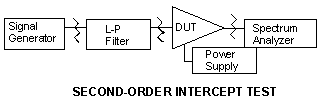
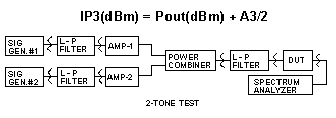
Q. Describe the procedure for measuring 2-tone 3-order intercept point of an amplifier.
A. Block diagram of a setup for measuring two-tone third- order intercept point is shown below.
If F1 & F2 are the frequencies of the two tones, then 2F1
F2 and 2F2 F1 are the third-order products. The set up
should ensure that second harmonic of F1 & F2 are at least 10
to 20 dB below the third-order products to be measured. Care also
should be taken to prevent F1 & F2 interaction and generation
of third-order products. Amplifiers 1 and 2 are selected such
that they have high directivity. This provides the desired
isolation of the generators. If A3 is the level of the
third-order product below the desired signal, then the output
third- order intercept point is given as
Last Updated: 12/17/1999